4 Out Of 11 As A Percentage
Arias News
May 10, 2025 · 5 min read
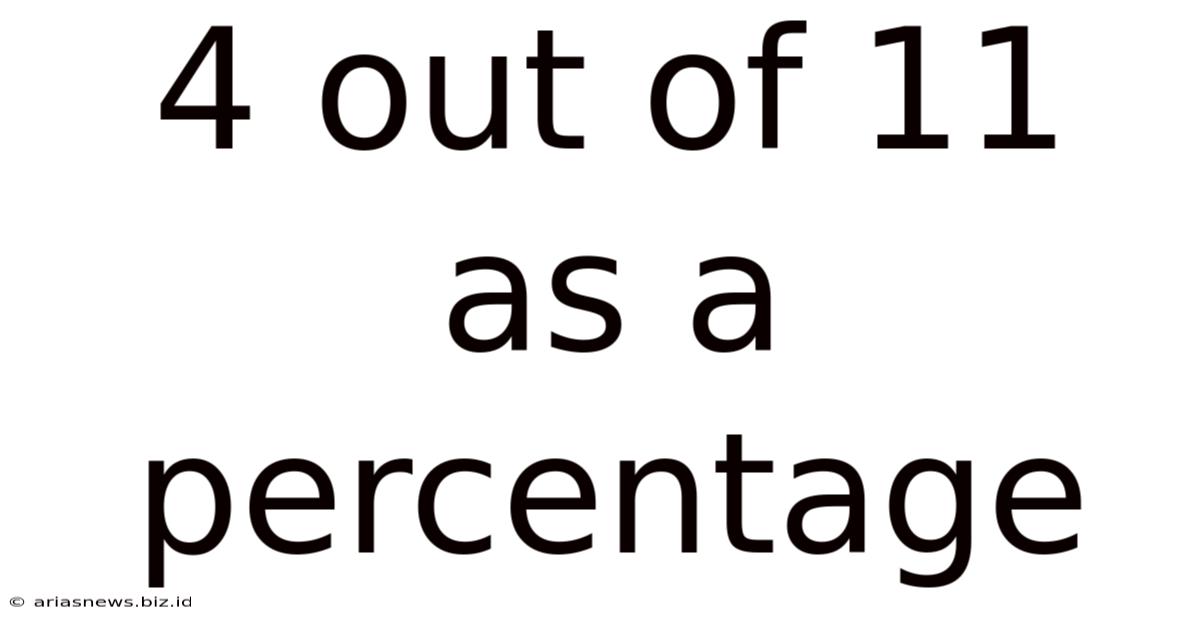
Table of Contents
4 Out of 11 as a Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with wide-ranging applications in various aspects of life, from everyday budgeting to complex statistical analysis. Understanding how to express fractions as percentages is crucial for interpreting data, making informed decisions, and communicating numerical information effectively. This comprehensive guide delves into the calculation of "4 out of 11 as a percentage," providing a step-by-step process, exploring different calculation methods, and highlighting practical applications.
Understanding the Basics of Percentages
Before we dive into the specific calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a portion of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Therefore, 50% means 50 out of 100, which is equivalent to the fraction ½ or 0.5 in decimal form.
The core concept lies in relating a part to a whole. In our case, "4 out of 11" represents the part (4) in relation to the whole (11). Our goal is to determine what percentage this part represents of the whole.
Method 1: The Direct Calculation Method
This method involves a straightforward conversion from fraction to percentage. We begin by expressing "4 out of 11" as a fraction:
4/11
To convert this fraction to a percentage, we need to make the denominator 100. We achieve this by multiplying both the numerator and the denominator by a number that will result in a denominator of 100. However, since 11 is not a divisor of 100, we'll use a different approach: we'll divide the numerator by the denominator and then multiply the result by 100.
-
Divide the numerator by the denominator: 4 ÷ 11 ≈ 0.3636
-
Multiply the result by 100: 0.3636 x 100 = 36.36
Therefore, 4 out of 11 is approximately 36.36%.
Method 2: Using Proportions
This method utilizes the concept of proportions to solve for the unknown percentage. We can set up a proportion as follows:
4/11 = x/100
Where 'x' represents the percentage we want to find. To solve for 'x', we cross-multiply:
11x = 400
Then, divide both sides by 11:
x = 400/11 ≈ 36.36
This confirms our previous result: 4 out of 11 is approximately 36.36%.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process considerably. Simply enter "4 ÷ 11" and then multiply the result by 100 to obtain the percentage. The calculator will directly provide the answer as approximately 36.36%.
Understanding Decimal Places and Rounding
The result we obtained (36.36%) is an approximation. The decimal representation of 4/11 (0.363636...) is a repeating decimal, meaning the digits "36" repeat infinitely. The number of decimal places we use depends on the required level of accuracy. For most purposes, two decimal places (36.36%) offer sufficient precision. However, in scenarios requiring higher accuracy, more decimal places might be necessary. It's crucial to understand the concept of rounding to avoid misinterpretations.
Practical Applications of Percentage Calculations
The ability to calculate percentages has countless practical applications across various domains:
1. Finance and Budgeting:
- Calculating discounts: Determining the final price after a percentage discount is a common application. For example, a 20% discount on a $100 item reduces the price by $20 (20% of $100).
- Interest rates: Understanding interest rates on loans, savings accounts, and investments is crucial for financial planning. Interest rates are expressed as percentages.
- Tax calculations: Calculating sales tax, income tax, or other taxes often involves percentage calculations.
2. Statistics and Data Analysis:
- Representing proportions: Percentages effectively represent proportions within a dataset, making it easier to understand and compare different segments.
- Calculating percentages of change: Determining the percentage increase or decrease in a value over time is essential for trend analysis and forecasting.
- Probability calculations: Percentages play a critical role in probability calculations, expressing the likelihood of an event occurring.
3. Everyday Life:
- Calculating tips: Determining the appropriate tip amount in a restaurant often involves calculating a percentage of the bill.
- Understanding survey results: Percentages are used extensively to represent the results of surveys and polls, making it easier to interpret the responses.
- Comparing prices: Comparing the prices of items with different quantities often necessitates converting prices to a percentage per unit.
Advanced Percentage Calculations: Beyond the Basics
While calculating "4 out of 11 as a percentage" covers the fundamental aspects, several more advanced percentage calculations exist:
1. Percentage Increase/Decrease:
Calculating the percentage change between two values involves finding the difference between the two values, dividing it by the original value, and then multiplying by 100. This formula is valuable for tracking changes in sales, profits, or other metrics over time.
2. Percentage of a Percentage:
Calculating a percentage of another percentage is a common scenario in complex calculations. For example, determining a discount on a discounted item involves calculating a percentage of a percentage.
3. Reverse Percentage Calculations:
Finding the original value before a percentage increase or decrease requires working backward. For instance, if an item costs $120 after a 20% increase, we can use algebraic techniques to determine the original price.
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations empowers you to confidently analyze data, make informed decisions, and effectively communicate numerical information. The simple calculation of "4 out of 11 as a percentage" serves as a foundation for understanding more complex percentage-based problems. By mastering the different methods discussed – the direct calculation, proportions, and calculator methods – and understanding the concepts of rounding and decimal places, you can confidently tackle various percentage-related challenges across multiple fields. Remember to practice regularly to solidify your understanding and further enhance your skills in this crucial area of mathematics. The applications of percentage calculations are vast and constantly relevant in our quantitative world.
Latest Posts
Latest Posts
-
Which Side Goes Up On A Winter Pool Cover
May 10, 2025
-
How To Copy Opacity Keyframes In Premier Pro
May 10, 2025
-
Names Of The Thieves Crucified With Jesus
May 10, 2025
-
If 40 Is Equal To The Fraction X 30
May 10, 2025
-
Average Shoe Size For A 5 11 Man
May 10, 2025
Related Post
Thank you for visiting our website which covers about 4 Out Of 11 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.